Input your answer in µm using 3 significant figures.
Wave Length λ = nm
Angle of the First Maximum θ = º
d = λ / sin θ
d = µm
d ≈ µm
11. What is the wavelength of light falling on double slits separated by 2.00 μm if the third-order maximum is at an angle of 60.0º ?
Double Slits Separation d = μm
Angle of the n = -Order Maximum θ = º
λ = d/n sin θ
λ = µm
λ ≈ µm
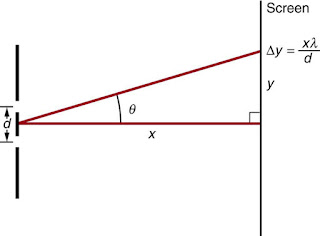
19. Calculate the distance between central and the first-order fringes for 633-nm light falling on double slits separated by 0.0800 mm, located 3.00 m from a screen.?
Input your answer in mm using 3 significant figures.
Wave Length λ = nm
Double Slits Separation d = mm
Distance to the Screen x = m
λ = d sin θ
λ/d = sin θ
Distance between Fringes Δy = x tan θ
Distance between Fringes Δy = mm
Δy ≈ mm
An approximate formula for small angles gives: Δy ≈ x λ / d = mm
27.4 Multiple Slit Diffraction
21. A diffraction grating has 2000 lines per centimeter. At what angle will the first-order maximum be for 520-nm-wavelength green light
Input your answer in degrees using 3 significant figures.
Density of the Lines (Number of Lines per Centimeter) n = cm-1
Wave Length λ = nm
The condition necessary to obtain constructive interference for a diffraction grating is
d sin θ = m λ, for m = 0, 1, –1, 2, –2, … (constructive), (27.11)
where d is the distance between slits in the grating, λ is the wavelength of light, and m is the order of the maximum.
Note that this is exactly the same equation as for double slits separated by d.
Solution:
d sin θ = λ
sin θ = λ / d
n = 1/d
sin θ = λ n
θ = arcsin (λ n)
Angle of the First-Order Maximum θ = °
θ ≈ °
27.5 Single Slit Diffraction
43. At what angle is the first minimum for 550-nm light falling on a single slit of width 1.00 μm?
Input your answer in degrees using 3 significant figures.
Hint:
Destructive Interference for a Single Slit:
D sin θ = m λ, form = 1, –1, 2, –2, 3, …(destructive), (27.21)
where
D is the slit width,
λ is the light’s wavelength,
θ is the angle relative to the original direction of the light, and
m is the order of the minimum.
Wave Length λ = nm
Slit Width D = μm
Solution:
D sin θ = λ
sin θ = λ / D
θ = arcsin (λ / D)
Angle of the First Minimum θ = °
θ ≈ °
27.5 Single Slit Diffraction
49. The light averaging 589 nm in wavelength falls on a single slit of width 7.50μm .
At what angle does it produces its second minimum?
Input your answer in degrees using 3 significant figures.
Hint:
Destructive Interference for a Single Slit:
D sin θ = m λ, form = 1, –1, 2, –2, 3, …(destructive), (27.21)
where
D is the slit width,
λ is the light’s wavelength,
θ is the angle relative to the original direction of the light, and
m is the order of the minimum.
Wave Length λ = nm
Slit Width D = μm
Order of the Minimum m =
Solution:
D sin θ = m λ
sin θ = m λ / D
θ = arcsin (m λ / D)
Angle of the m-order Minimum θ = °
θ ≈ °
>
No comments:
Post a Comment